Funny Serious Stuff by Massimo Guiggiani
I believe that playing and working are not much different. Both challenge our mind, both require our attention, both can be rewarding.
In this page you will find some of my favourite games, tricks, experiments. Some of them are original, some others I found somewhere. I hope you will enjoy at least a few of them. Of course, any suggestion and/or criticism is more than welcome.
Suggestions on how not to mishandle mathematical formulae
Learn how to write properly mathematical equations and formulae. Communication skill should not be underestimated.
Amazing vibrations
A really interesting video on the mechanics of vibrations.
Find what comes next in the sequence (0, 1, 2, 720!, …)
Well, it is easy. 720 = 6!, and 6 = 3!. Therefore 720! = 3!!! (three, three times factorial). Then realize that 0 = 0, 1 = 1!, 2 = 2!! (remember 0! = 1).
Here is the sequence 0, 1!, 2!!, 3!!!, 4!!!!, …
3!!! has 1746 digits. 4!!!! is
 |
Stacking blocks
If you stack up bricks (or dominoes) one on top of the other, what is the greatest horizontal distance that can be covered before the stack tips over? Note that we assume there is no glue.
Well, this is a classical problem. But I found that the stack hides a small great secret. In fact it tells us how to build the Nepero (Napier) number by using bricks! This is shown in the following picture. You need 1 = e^0 bricks to achieve one unit of horizontal distance (half brick). Then, you have to add e = e^1 bricks (about 2.7) for getting one additional horizontal unit. For getting another unit, you need e^2 bricks, and so on. Isn't that nice?
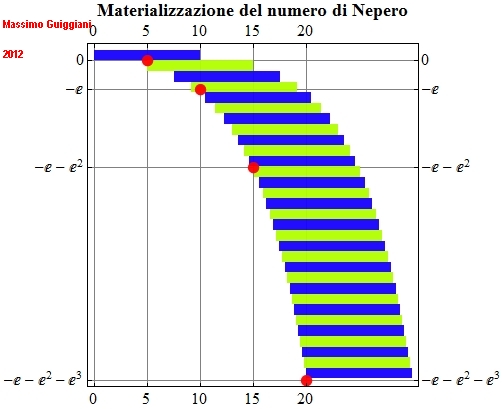 |
A huge pile of paper
A sheet of paper has a thickness of about 0.1 mm. Then, two sheets make 0.2 mm. Let us double them again: we have four sheets and a thickness of 0.4 mm. Let us double this pile of paper 51 times. How thick does it get? Well, it is very thick indeed…about 1.5 the distance between the Earth and the Sun. That is the power of exponential growth.
Flip a coin
Coin flipping, coin tossing, cross and pile, or heads or tails (H or T) is the practice of throwing a coin in the air to choose between two alternatives, sometimes to resolve a dispute between two parties. It is a form of sortition which inherently has only two possible and equally likely outcomes.
Everybody knows that H and T are equally probable. Well, let us state it more precisely. That means that the difference H - T tends to zero the more times we flip the coin…Or not?? Actually not!!
Instead, it is true that the ratio H/T (or viceversa) tends to 1, and does it very quickly.
The ratio tends to 1, but that does not imply at all that the difference tends to 0. Don't believe it? Just consider the limit for x tending to infinite of the ratio and of the difference of f(x) = (x + x^2) and g(x) = x^2…
The Botafumeiro
The Botafumeiro is a famous thurible found in the Santiago de Compostela Cathedral. Incense is burned in this swinging metal container, or “incensory”. The name “Botafumeiro” means “smoke expeller” in Galician.
The Botafumeiro is suspended from a pulley mechanism in the dome on the roof of the church. The current pulley mechanism was installed in 1604. The thurible is tied to the rope with elaborate knots. The censer is pushed initially to start its motion. Eight red-robed tiraboleiros pull the ropes, producing increasingly large oscillations of the censer. The turible's swings almost reach the ceiling of the transept.
The question is “How do you have to pull the rope to get increasingly large oscillations?” Try yourself here!
Orthogonal or not orthogonal: that is the question
Coming soon.
The ring and the chain, or “Is topology just an opinion?”
Watch this short video, and then this video in slow motion.
Magic spinning top
Static equilibrium does not necessarily mean dynamic equilibrium. Watch this short video.